what are vector quantities
VECTORS AND SCALARS
Certain physical quantities described by a numerical value alone (with units specified) and are added according to the ordinary
are
completely
rules of algebra. As an example the mass of a systemn is described by saying that it is 5 kg. If two bodies one having a mass of 5 kg and the other having a mass of 2 kg are added together tè
o make a composite system,
the total mass of the system becomes 5 kg + 2 kgg = 7 kg. Such quantities are called scalars.
2 kg
The complete description of certain physical quantities requires a numerical value (with units specified) as well as a direction in space. Velocity of a particle is an example of this kind. The magnitude of velocity is represented by a number such as 5 m/s and tells us how fast a particle is moving. But the description of velocity becomes complete only when the direction of velocity is also specified. We can represent this velocity by drawing a line parallel to the velocity and putting an arrow showing the direction of velocity.
We can decide beforehand a particular length to represent 1 m/s and the length of the line representing
a velocity of 5 m/s may be taken as 5 times this unit
length. Figure (2.1) shows representations of several velocities in this scheme. The front end (carrying the arrow) is called the head and the rear end is called the tail.
Further, if a particle is given two velocities simultaneously its resultant velocity is different from the two velocities and is obtained by using a special rule. Suppose a small ball is moving inside a long tube at a speed 3 m/s and the tube itself is moving in the room at a speed 4 m/s along a direction perpendicular to its length. In which direction and how fast is the ball moving as seen from the room?
 |
Figure (2.2) shows the positions of the tube and the ball at t = 0 and t = 1 s. Simple geometry shows that the ball has moved 5 m in a direction 0 = 53° from the tube. So the resultant velocity of the ball is 5 m/s along this direction. The general rule for finding the resultant of two velocities may be stated as follows.
Draw a line AB representing the first velocity with
B as the head. Draw another line BC representing the second velocity with its tail B coinciding with the head of the first line. The line AC with A as the tail and C the
resultant velocity.
as the head represents the
Figure (2.3) shows the construction.
The resultant is also called the sum of the two
velocities. We have added the two velocities AB and
BC and have obtained the sum AC. This rule ot
and
addition is called the "triangle rule of addition".
The physical quantities which have magnitude and direction and which can be added according to the triangle rule, are called vector quantities. Other
is
Other
examples of vector quantities are force, linear momentum, electric field, magnetic field etc.
of
linear
The vectors are denoted by putting an arrow over
the symbols representing them. Thus, we write AB
BC etc. Sometimes a vector is represented by a single
letter such as v, F etc. Quite often in printed books the vectors are represented by bold face letters like
AB, BC, v, f etc.
If a physical quantity has magnitude as well as direction but does not add up according to the triangle rule, it will not be called a vector quantity. Electric current in a wire has both magnitude and direction but there is n0 meaning of triangle rule there. Thus, electric current is not a vector quantity.
2.2 EQUALITY OF VECTORS
(Two vectors (representing two values of the same physical quantity) are called equal if their magnitudes and directions are same. Thus, a parallel translation of a vector does not bring about any change in it.
2.3 ADDITION OF VECTORS
The triangle rule of vector addition is already described above. If a and b are the two vectors to be added, a diagram is drawn in which the tail of b coincides with the head of a. The vector joining the tail of a with the head of b is the vector sum of a and
b. Figure (2.4a) shows the construction. The same rule
may be stated in a slightly different way. We draw the vectors a and b with both the tails coinciding (figure 2.4b). Taking these two as the adjacent sides
Example to follow














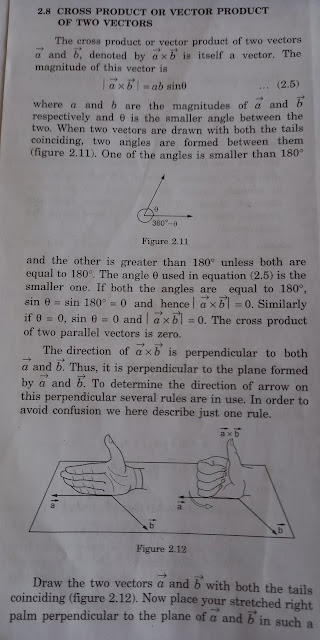






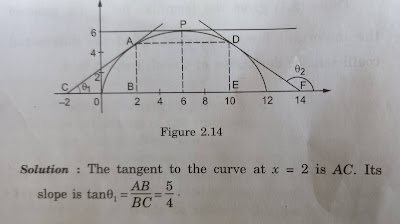




Comments
Post a Comment